Effective Nuclear Charge Definition Chemistry
1.1.2: Effective Nuclear Accuse
- Page ID
- 195506
Constructive Nuclear Charge (\(Z_{eff}\))
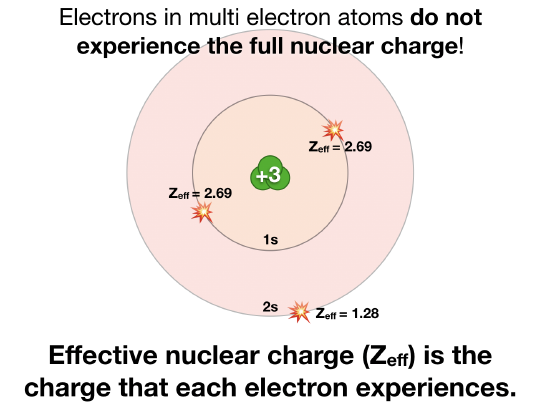
Figure \(\PageIndex{one}\). Effective nuclear accuse in a Li atom. (CC-BY-NC-SA; Kathryn Haas)
The platonic gas law is easy to think and apply in solving problems, every bit long as you go the proper values a
According to Coulomb'due south law, the attraction of an electron to a nucleus depends only on three factors: the charge of the nucleus (+Z), the charge of the electron (-1), and the altitude between the two (\(r\)). Coulomb's law works well for predicting the energy of an electron in a hydrogen cantlet (H has but two particles: i nucleus and one electron). It as well works for hydrogen-like atoms: whatever nucleus with exactly i electron (a He+ ion, for example, has i electron). However, Coulomb's constabulary is bereft for predicting the energies of electrons in multi-electron atoms and ions.
Electrons inside a multi-electron atom interact with the nucleus and with all other electrons. Each electron in a multi-electron atom experiences both attraction to the nucleus and repulsion from interactions with other electrons. The presence of multiple electrons decreases the nuclear attraction to some extent. Each electron in a multi-electron atom experiences a different magnitude of (and attraction to) the nuclear charge depending on what specific subshell the electron occupies. The corporeality of positive charge experienced by whatever individual electron is the effective nuclear accuse (\(Z_{eff}\)). **
For case, in lithium (Li), none of the three electrons "experience" the total +3 charge from the nucleus (see Cartoon). Rather, each electron "feels" a \(Z_{eff}\) that is less than the actual Z and that depends on the electron's orbital. The bodily nuclear charge in Li is +three; the 1s electrons experience a \(Z_{eff}\) =+2.69, and the 2s electron experiences a \(Z_{eff}\) = 1.28. In general, cadre electrons (or the electrons closest to the nucleus), "experience" a \(Z_{eff}\) that is close to, but less than, the bodily nuclear accuse (Z). On the other hand, outer valence electrons experience a \(Z_{eff}\) that is much less than Z.
In summary:
- Core electrons: \(Z^* \lessapprox Z\)
- Valence electrons: \(Z^* \ll Z\)
**You lot will as well see \(Z_{eff}\) represented as \(Z^*\): specifically in the section in which y'all reviewed Periodic Trends, the symbol \(Z^*\) was used.
Slater'southward rules for estimating \(Z_{eff}\)

Figure \(\PageIndex{ii}\). Diagram illustrating effective nuclear charge according to Slater's rules.
The \(Z_{eff}\) tin be estimated using a number of different methods; probably the best known and most commonly used method is known as Slater's Rules. Slater developed a set of rules to estimate \(Z_{eff}\) depending on how many other electrons exist in the atom and on the orbital location of the electron-of-interest. These two factors are important determinants in shielding (see next section), and they are used to summate a shielding constant (\(\sigma\)) used in Slater's formula:
\[Z_{eff}=Z-\sigma\]
where Z is the actual nuclear charge (the atomic number) and \(Z_{eff}\) is the effective nuclear charge.
To calculate \(\sigma\), we will write out all the orbitals in an atom, separating them into "groups". Each alter in shell number is a new group; s and p subshells are in the same group but d and f orbitals are their own group. You write out all the orbitals using parentheses until you get to the group of the electron-of-interest, like this:
(1s)(2s,2p)(3s,3p)(3d)(4s,4p)(4d)(4f)(5s,5p) etc.
**Critical: The orbitals must be written in club of increasing energy!
- Electrons in the aforementioned Group(): Each other electron (non counting the electron-of-interest) in the aforementioned grouping () every bit the chosen electron, contributes 0.35 to \(\sigma\).
Conceptually, this ways electrons in the same grouping shield each other 35%. - Electrons in Groups() to the left:
- If the electron-of-interest is in a d or f subshell, every electron in groups () to the left contributes 1.00 to \(\sigma\).
Conceptually, this ways that d and f electrons are shielded 100% by all electrons in the same shell with a smaller value of \(l\), likewise every bit all electrons in lower shells (\(n\)). - If the electron-of-involvement is in an s or p subshell, all electrons in the side by side lower vanquish (northward - 1) contribute 0.85 to \(\sigma\). And all the electrons in even lower shells contribute 1.00 to \(\sigma\).
Conceptually, this ways that s and p electrons are shielded 85% past the electrons ane trounce lower, and 100% by all electrons in shells due north - 2 or lower.
- If the electron-of-interest is in a d or f subshell, every electron in groups () to the left contributes 1.00 to \(\sigma\).
- 1s electrons: \(\sigma\) of a 1s electron is just (\sigma=0.3\), no matter the element.
A video explaining how to use Slater's Rules
What is the \(Z_{eff}\) experienced past the valence electrons in the 3 isoelectronic species: fluorine anion (F-), neutral neon atom (Ne), and sodium cation (Na+)?
Solution
Each species has 10 electrons, and the number of core electrons is 2 (10 total electrons - 8 valence), but the effective nuclear charge varies because each has a different atomic number (Z). The judge \(Z_{eff}\) tin can be found with Slater'due south Rules. For all of these species, we would calculate the same sigma value:
Calculating \(\sigma\): (1s)(2s,2p), \(\sigma = ii(0.85) + 7(0.35) = one.7 + two.45 = iv.15 \)
Fluorine anion: \(Z_{eff}=9-\sigma = 9 - four.xv = 4.85\)
Neon atom: \(Z_{eff}=x-\sigma = x - 4.15 = 5.85\)
Sodium Cation: \(Z_{eff}=11-\sigma = 11 - four.fifteen = 6.85\)
So, the sodium cation has the greatest effective nuclear accuse.
Calculate Zeff for a 3d-electron in a zinc (Zn) cantlet.
- Answer
-
Write out the relevant orbitals: (1s)(2s,2p)(3s,3p)(3d)
(4s)Notice that although 4s is fully occupied, we don't include it because in Zn, 4s is college in energy than 3d, and is thus to the right of the d electrons we are looking at. The electron-of-involvement is in 3d, so the other 9 electrons in 3d each contribute 0.35 to the value of S. The other 18 electrons each contribute one to the value of South.
\(S=eighteen(1)+9(0.35)=21.15\)
\(Z_{eff}=30-21.fifteen=8.85\)
And then, although the nuclear charge of Zn is xxx, the 3d electrons only experience a \(Z_eff \approx 8.85\)!
"Best" values for \(Z_{eff}\)
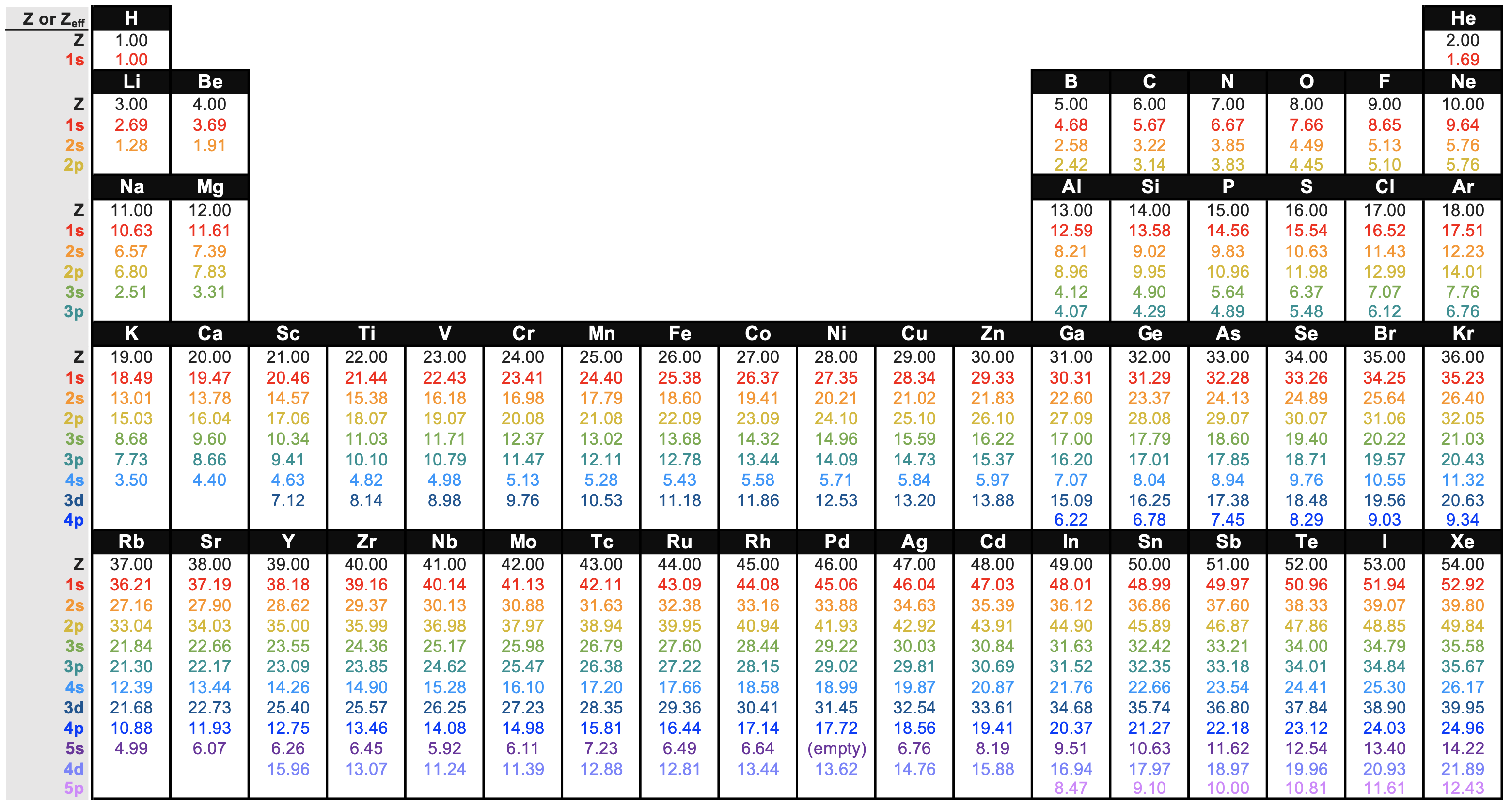
Slater's rules are a set of unproblematic rules for predicting \(\sigma\) and \(Z_{eff}\) based on empirical prove from quantum mechanical calculations. In other words, the \(Z_{eff}\) calculated from Slater's rules are approximate values. The values considered to be the nigh accurate are derived from quantum mechanical calculations directly. You tin can find these values in a nice chart on the Wikipedia commodity of Effective Nuclear Charge. I've recreated the chart in Figure \(\PageIndex{3}\) for convenience:
\(Z_{eff}\) modulates attraction
When valence electrons feel less nuclear charge than core electrons, unlike electrons experiencing different magnitudes of attraction to the nucleus. A modified form of Coulomb's Police is written below, where \(east\) is the charge of an electron, \(Z_{eff}\) is the effective nuclear charge experienced by that electron, and \(r\) is the radius (distance of the electron from the nucleus).
\[ F_{eff}=1000 \dfrac{Z_{eff}e^two}{r^2}\]
This formula would advise that if nosotros tin guess \(Z_{eff}\), and then nosotros can predict the attractive strength experienced by, and the energy of, an electron in a multi-electron cantlet, like Li.
The allure of the nucleus to valence electrons determines the atomic or ionic size, ionization free energy, electron affinity, and electronegativity. The stronger the attraction, and the stronger \(Z_{eff}\), the closer the electrons are pulled toward the nucleus. This in turn results in a smaller size, higher ionization free energy, higher electron affinity, and stronger electronegativity.
Full general Periodic Trends in \(Z_{eff}\)
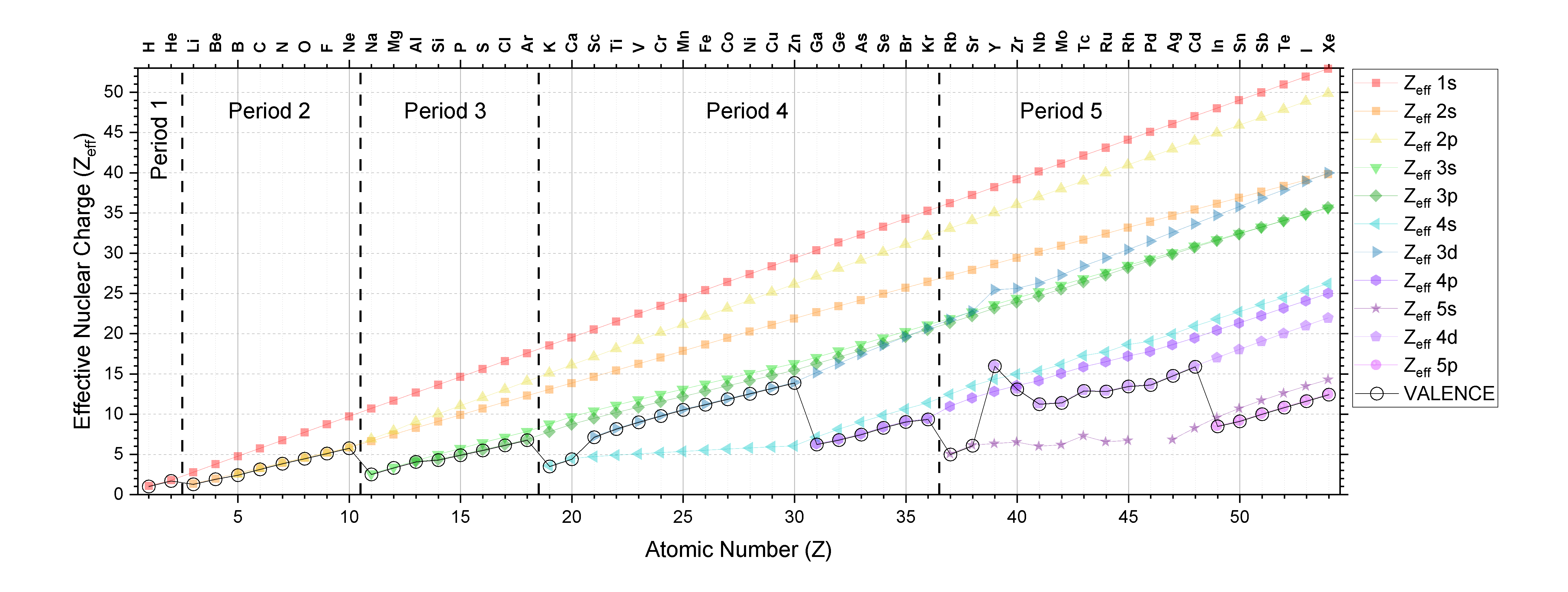
Shut inspection of Effigy \(\PageIndex{three}\) and analysis of Slater's rules betoken that at that place are some predictable trends in \(Z_{eff}\). The information from Figure \(\PageIndex{3}\) is plotted beneath in Effigy \(\PageIndex{four}\) to provide a visual aid to the discussion below.
Trends in \(Z_{eff}\) for electrons in a specific shell and subshell
The \(Z_{eff}\) for electrons in a given beat out and subshell by and large increases equally diminutive number increases; this trend holds true going across the periodic table and downwardly the periodic table. Convince yourself that this is truthful for any subshell by examining Effigy \(\PageIndex{4}\).
- Do yous detect any exceptions to this full general trend?
-
Inspection of figure \(\PageIndex{four}\) should confirm for you that the \(Z_{eff}\) increases as Z increases for electrons in any subshell (like the 1s subshell for case, which is plotted to a higher place as a cerise line with square points). You can see this trend as the positive slope in each series. There is one obvious exception in Menstruum 5 in elements 39 (Y) to 41 (Nb; the \(Z_{eff}\) of 4s actually decreases across these iii elements as atomic number increases. There is also an exception between Y and Zr in the 3d subshell, and between Tc and Ru in the 5s subshell.
For valence electrons:
It is useful to empathize trends in valence \(Z_{eff}\) considering the valence \(Z_{eff}\) determines atomic/ionic properties and chemic reactivity. The trends in the valence \(Z_{eff}\) are not elementary because every bit atomic number increases, the valence trounce and/or subshell also changes. The valence \(Z_{eff}\) is indicated in Figure \(\PageIndex{iv}\) as a black line with open circles.
Down the tabular array: As nosotros go downwardly a cavalcade of the periodic table, the valence \(Z_{eff}\) increases. This is a simple trend because type of subshell is consequent and in that location is an increase only in beat out and in atomic number, Z. This trend is best illustrated by inspection of Figure \(\PageIndex{3}\).
Across the table: the tendency depends on beat and subshell.
Periods 1-three (s and p merely): As we become across the table in periods 1-3, the vanquish stays constant as Z increases and subshell changes from s to p. In these periods, there is a gradual increase in valence \(Z_{eff}\) as nosotros move across whatsoever of the first iii periods.
Periods iv and 5 (south, p, and d): Now we have some more complex trends because valence subshell and shell are changing as we increase in atomic number. Discover that the valence \(Z_{eff}\) generally increases going across a period equally long equally subshell isn't changing; the exception is inside the 4d subshell (elements 39-44 or Y-Ru). In general, going from \((n)s\) subshell to \((north-1)d\) subshell, in that location a relatively large increase in valence \(Z_{eff}\). And in going from \((northward-one)d\) subshell to \((north)p\) subshell, there is a relatively large decrease in \(Z_{eff}\).
From ane period to another: From Effigy \(\PageIndex{4}\), we can run into that as nosotros increase Z by one proton, going from 1 period to the next, in that location is a relatively large decrease in \(Z_{eff}\) (from Ne to Na, for example). This is because equally Z increases by a small interval, the beat number increases, and so the electrons in the valence crush are much farther from the nucleus and are more shielded by all the electrons in the lower shell numbers.
Exercises
i. Compare trends in \(Z_{eff}\) and atomic size. Explain how and why atomic size depends on \(Z_{eff}\).
ii. Compare trends in \(Z_{eff}\) and ionization energy. Explain how and why ionization energy depends on \(Z_{eff}\).
- Answer
-
i. Equally \(Z_{eff}\) increases, the valence electrons are pulled in tighter to the nucleus resulting in a small radius for atoms.
ii. As \(Z_{eff}\) increases, the distance between the valence electrons and the nucleus decreases. This creates a stronger force holding the valence electrons, and thus requires a higher ionization energy to remove a valence electron.
Effective Nuclear Charge Definition Chemistry,
Source: https://chem.libretexts.org/Courses/Saint_Marys_College_Notre_Dame_IN/CHEM_342%3A_Bio-inorganic_Chemistry/Readings/Week_1%3A_Analysis_of_Periodic_Trends/1.1%3A_Concepts_and_principles_that_explain_periodic_trends/1.1.2%3A_Effective_Nuclear_Charge
Posted by: westbrookwhanderharty.blogspot.com


0 Response to "Effective Nuclear Charge Definition Chemistry"
Post a Comment